|
1.
|
Open a new sketch, and construct
a triangle. Label the vertices A, B, and C. |
|
2.
|
Construct all three altitudes. To do this, select
one vertex and the opposite side. From the Construct menu, choose Perpendicular
Line. Repeat these steps with the other two vertices. |

|
|
3.
|
The three altitudes should be concurrent. Why?
Sketchpad cannot define the intersection point of three lines, so select
any two of the altitudes. From the Construct menu, choose Point At Intersection.
Label the orthocenter D. |
|
4.
|
Drag the triangle vertices, and
observe the position of the orthocenter. Under what conditions is it on
the interior of the triangle? When is it on the exterior? When is it on
a side of the triangle? When is it on a vertex? |
|
5.
|
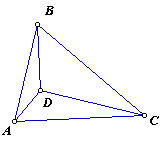
Construct the line segments DA,
DB,
and DC. Hide the altitude lines. There are now three triangles on
the sketch, ΔABC, ΔDAB,
ΔDBC,
and ΔDCA. You have already constructed
the orthocenter of ΔABC. Where are the
orthocenters of the other triangles? |
|
|
6.
|
Before going any further, select
all four points and all six sides. From the Edit menu, choose Action Button,
Hide/Show. The two buttons that appear on the screen will help you organize
the drawing if it gets cluttered, which it will. It also would help to
give the segments some color. Select the six segments. From the Display
menu, choose Color, and select a color. |
|
7.
|
Construct the midpoints of all six
segments. To do this, select the segments, and from the Construct menu,
choose Point At Midpoint. |
|
8.
|
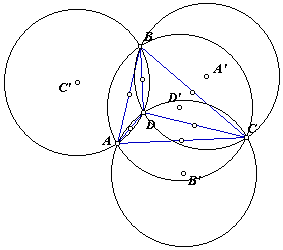
Use the midpoints to construct the
perpendicular bisectors of the sides; then use the perpendicular bisectors
to construct the circumcenters of all four triangles. Use the text tool
to label the four circumcenters. The circumcenter of ΔABC
should
be labeled D', the circumcenter of ΔDAB
should
be labeled C', and so on. The reason for this will become clear
later. |
|
9.
|
Hide the bisectors, and use the circumcenters
to construct the circumcircle of each of the four triangles. What do you
observe about the circles? Can you explain their relation? Those circles
might be useful later. Select all four of them, and create Hide/Show buttons.
Hide the circles for now. |
|
|
10.
|
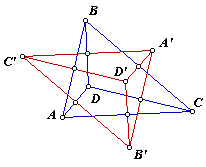
From the Display menu, choose Color. Select
some color other than the last one you used. Now construct line segments
connecting each pair of the points in A', B', C',
and D'. What do you observe? |
|
11.
|
This new system appears to be congruent
to the first one. Can you support this with measurements? Can you prove
it? |
|
|
12.
|
In fact, the systems are congruent.
They also are 180° rotations of each other. A 180° rotation is
the equivalent of a point reflection. Construct lines between corresponding
points (A and A', B and B', etc). They should
intersect at the reflection point. |
|
13.
|
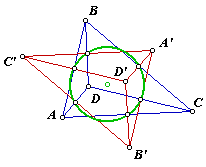
The reflection point has another
special property. Choose the circle tool from the tool bar. Click on the
reflection point and drag to one of the segment midpoints. This is called
the nine-point circle. It includes six of the points identified
on this sketch. See if you can find the other three (actually, six). How
does the nine-point circle compare with the circumcircles that were constructed
earlier? |
|
|
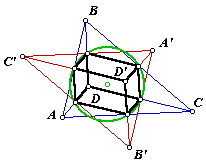
14.
|
There is still more. If ΔABC is
not acute, drag the vertices so that it is. On the Display menu, set the
line style to draw a thick, black line. From the tool bar, choose the segment
tool. Go around the circle in order, connecting each pair of adjacent midpoints
with a line segment. Next, connect point D with each of its adjacent
midpoints. Do the same with D'. The black segments have drawn a
projection of a rectangular solid. Drag the vertices again. Why does this
not work when ΔABC is obtuse? |
|
Go back to the orthocentric system again. When point A is dragged,
points B and C do not move, but point D does. What
shape is traced by point D when point A moves along a line?
What happens when Point A moves along a circle?